Hockey-Stick theorem

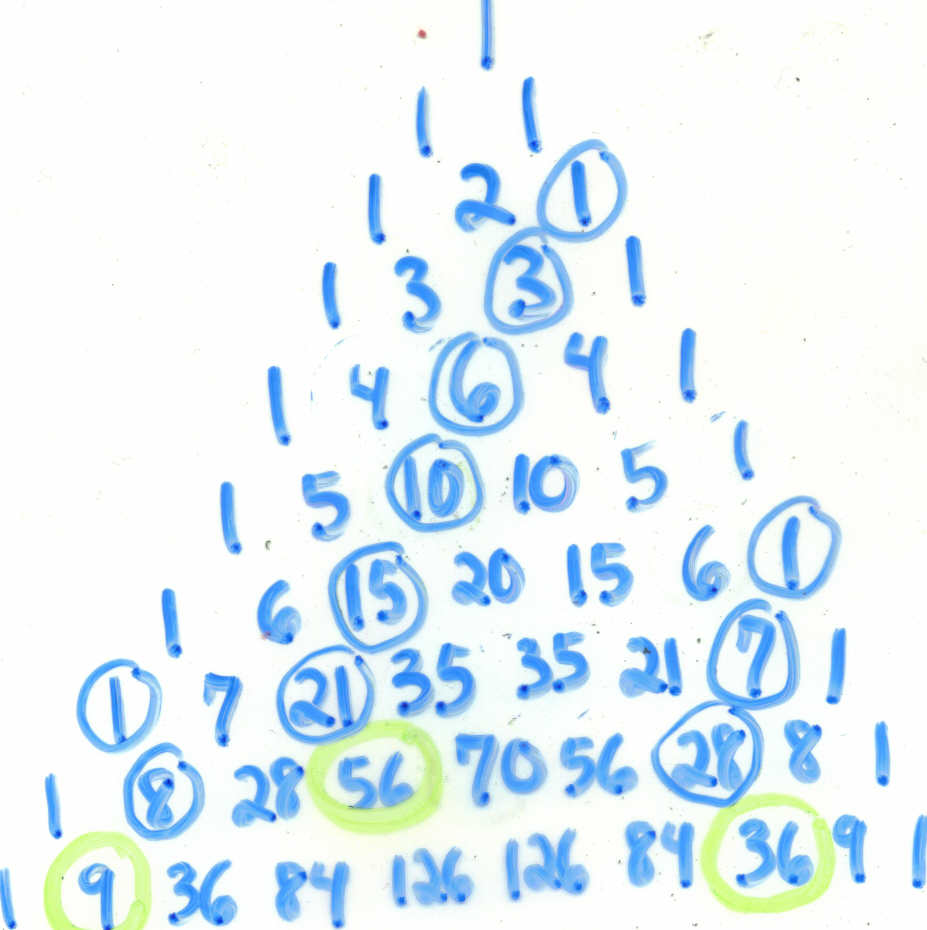
Now we know that for every number in the triangle, the addition of the two numbers directly above it constitute its sum. Therefore, we can expand the original two numbers to eventually form a figure that looks like a hockey stick (above).

For example, in the figure above, 56 (highlighted in green) is the sum of 35 and 21. But 35 is the sum of 15 and 20. And 20 is the sum of 10 and 10, and so on. Once we have exhausted our possible expansions with this technique, the figure looks like a hockey stick.

Therefore, the base of the stick is the sum of all the numbers located in the handle of the stick.

We can also have the handle pointing the other direction. In the example above, 9 is the sum of 8 and 1, and this particular stick has a different orientation than the first one I described.